Conservation Equations in Fluid Dynamics
- Anand Zambare
- Jul 23, 2021
- 5 min read
In the previous article, we have discussed the shear stress and fundamentals of fluid dynamics. In case if you have missed it please find it here.
Table of Content
6) History
7) Summary

Introduction to the first principle:
The topic of this article is conservation equations of fluid flows and their role in fluid dynamics. From the basics of fluid mechanics we have understood that there are three fundamental laws in nature i.e. law of conservation of 1) Mass 2) Momentum 3) Energy. These laws are used by physicists in different subjects under different names. For example, if we consider the first law of thermodynamics then we can simply state that it is the law of conservation of energy (Stated differently for open as well as closed systems).
In the case of Fluid Dynamics, the law of conservation of mass is also known as the “Continuity equation.” We can say that with respect to fluid mechanics these laws are the first principles of the subject. Now you must be wondering what is the first principle?
The first principles are nothing but the most fundamental laws for the given topic/subject/phenomena which do not involve assumptions i.e. they are true for any given scenario.
So for fluid mechanics, these are the most fundamental laws. Now the next and very important question to be answered is what is the need for such laws? The answer is quite simple, if we want to understand what is exactly happening in the phenomena and predict what will happen when we change some input parameters then we need to use these laws.
Let us consider a scenario in which we are looking at the melting of ice first and boiling of water. If we want to understand these two phenomena we can apply the principle of conservation of mass and energy to understand and predict it. As there is no dynamic motion involved in this, there is no need for the implementation of momentum law. So we have defined the need physically. Mathematically speaking the application of conservation laws can be used to formulate the governing equations for the given phenomena.
The Conservation of Mass:
Let’s understand the mathematical use of these conservation principles. Consider a differential volume of fluid as shown in the figure. Let us say the mass of an element is ‘m’ the density is ‘rho’ and volume is ‘v’. We can define mass m as
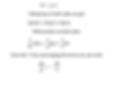
The above fundamental equation can mathematically represent the conservation of mass equation. Given the equation of state followed by the fluid, we can mathematically formulate the given problem. Similarly, we can use the conservation of momentum and energy to formulate the given situation.
The Mathematical Form of All Conservation Laws:

Where rho is density, p is pressure, v is velocity, b is the body force, T is temperature, Pr is Prandtl number and tau is shear stress.
The equations stated above are the general forms of the conservation equations for mass, momentum, and energy respectively. There is the use of indices such as i and j such indices are used to denote the presence of specific directions and they are free indices where each of them can take any value between 1, 2, and 3 representing the x, y, and z-direction respectively for the rectangular coordinate system.
So far we have seen, the forms of conservation equations. The role played by them physically as well as mathematically. Now we will be discussing more the mathematical part of conservation equations.
The Navier’s Equilibrium Equation:
This equation is a general form of the conservation of momentum. The left-hand side is representing the acceleration terms and the right-hand side represents the surface and body forces.
The important part of the right-hand side is the shear stress term and we have already seen that the shear stress can be thought of as two components i.e. Deviatoric and Hydrostatic. Also, we have already seen that assuming the Homogenous Newtonian fluids we can form a relationship between the shear stress and shear strain. This leads to the formation of Navier-Stokes equations. Let’s look at the X-component of the Navier-Stokes equation now.

Where mu represents dynamic viscosity, D is used to represent the total derivative or material derivative. This is valid for Homogenous, Newtonian, Compressible, or Incompressible fluids.
It is the nonlinear PDE that will be coupled with continuity and Energy equations if there are any energy interactions. We have already discussed the solution and role of CFD in this incase if you have missed it you can check it here.
The Energy Equation:
The energy equation is seldom discussed in the fluid mechanics area because most of the time there are no energy interactions involved (or to be precise we consider it in that way) but when there are energy interactions then there is the involvement of the energy equations.
Solution of Energy equations over the domain gives us the temperature distribution in the domain which can be used to find the temperature gradients and various heat as well as energy interactions.
For dealing with such problems, we have 1) Continuity equation 2) Momentum equation 3) Energy equation. So basically we have 5 equations (1 continuity + 1 energy + 3 momentum) and 7 unknowns that are density, pressure, 3 velocity components, temperature, and energy. So to close the system of equations we need two more equations.
One more equation comes from the equation of the state and another comes from the relationship between energy and temperature as some function of pressure (Thermodynamic part) also known as the “Caloric equation of state.” So this is how we can get the closure of the equations and no. of unknowns.
Now we can say that given a situation it is possible for us to apply conservation principles to it and form the mathematical equations or model of that situation. Further, as they are highly nonlinear coupled partial differential equations we have to solve them numerically to find its solution and get the desired results.
History :
The development of Conservation equations started way back when people like Leonhard Euler, Poisson started attacking the problems from fluid mechanics. The big idea for the general conservation principle came from Leibniz - Reynold's Theorem which is more known as RTT i.e. Reynold's Transport Theorem.
RTT is a generalization of Leibniz's Integral rule i.e. rule of differential under the integral sign. Osborne Reynold's spent his entire life doing research at the University of Manchester. His findings are well known. Even high schoolers are aware of Reynold's number which is the distinguishing factor between laminar and turbulent flows.
His work in turbulence is known widely and we all are aware of the deduction of Reynold's Averaged Navier Stokes equations (RANS) used in turbulence modeling. It will be an injustice to Sir Daniel Bernoulli if we talk about Conservation equations but don't talk about Bernoulli's equations. His contributions in the field are highly useful especially when flows are irrotational without any energy interactions.
Summary:
There are three fundamental laws in physics which we use as a first principle in fluid mechanics they are laws of conservation of mass, momentum, and energy. First-principles are nothing but laws without any assumptions leading to any particular cases.
Application of Conservation laws gives physical as well as mathematical sense to the problem. To formulate the problem mathematically it is necessary to use the appropriate form of each conservation equation.
When there is the involvement of energy interactions we need to consider the energy equation also for finding the solution to the problem. Solving the energy equation gives us temperature distribution in the domain.
For closing the no. of equations and unknowns we have to use the thermodynamic relations like the equation of state and caloric equation of state.
I hope all the readers have enjoyed the journey of fluid dynamics so far. We have discussed all the background work required for further discussions like Finite Volume Method (FVM), Finite Element Method(FEM), etc. See you all in the next article. Thank you!
.png)
